
 |
| Home | Teacher | Parents | Glossary | About Us | |||||||||||
|
|
|||||||||||
|
|
In this lesson we look at some properties that apply to all real numbers. If you learn these properties, they will help you solve problems in algebra. Let's look at each property in detail, and apply it to an algebraic expression.
#1. Commutative
properties
The commutative
property of addition says that we can add numbers in any order. The commutative
property of multiplication is very similar. It says that we can multiply numbers
in any order we want without changing the result.
addition
5a + 4 = 4 + 5a
multiplication
3 x
8 x
5b = 5b x
3 x
8
#2. Associative
properties
Both
addition and multiplication can actually be done with two numbers at a time.
So if there are more numbers in the expression, how do we decide which two
to "associate" first? The associative property of addition
tells us that we can group numbers in a sum in any way we want and still get
the same answer. The associative property of multiplication tells us that
we can group numbers in a product in any way we want and still get the same
answer.
addition
(4x + 2x) + 7x = 4x + (2x + 7x)
multiplication
2x2(3y) = 3y(2x2)
#3.
Distributive property
The distributive
property comes into play when an expression involves both addition and multiplication.
A longer name for it is, "the distributive property of multiplication
over addition." It tells us that if a term is multiplied by terms in
parenthesis, we need to "distribute" the multiplication over
all the terms inside.
2x(5 + y) = 10x + 2xy
Even though order of operations says that you must add the terms inside the parenthesis first, the distributive property allows you to simplify the expression by multiplying every term inside the parenthesis by the multiplier. This simplifies the expression.
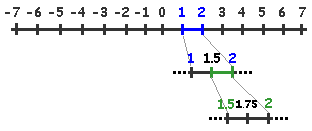
#4. Density
property
The density property
tells us that we can always find another real number that lies between any
two real numbers. For example, between 5.61 and 5.62, there is 5.611, 5.612,
5.613 and so forth.
Between 5.612 and 5.613, there is 5.6121, 5.6122 ... and an endless list of other numbers!
#5. Identity
property
The identity
property for addition tells us that zero added to any number is the number
itself. Zero is called the "additive identity." The identity property
for multiplication tells us that the number 1 multiplied times any number
gives the number itself. The number 1 is called the "multiplicative identity."
Addition
5y + 0 = 5y
Multiplication
2c × 1 = 2c
| Homework Help | Algebra | The Basics of Algebra | |||
|
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||