Constant Rule

Home | Teacher | Parents | Glossary | About Us

	Resources
	· Cool Tools · Formulas & Tables · References · Test Preparation · Study Tips · Wonders of Math
	Search

Proof: Constant Rule

(Math | Calculus | Derivatives | Identities | Constant Rule)

c f(x) = c (d/dx)

f(x)

Proof of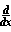

c f(x) = c (d/dx)

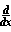f(x) from the definition

We can use the definition of the derivative:

f(x) = lim
^d-->0 f(x+d)-f(x)
d

Therefore, 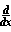(d/dx)

c f(x) can be written as such:

c f(x) =

lim
^d-->0 cf(x+d) - cf(x)
d

c lim
^d-->0 f(x+d) - f(x)
d

= c * f(x)