 c f(x) =
c f(x)
[ f(x) + g(x)
] = f(x) + g(x)
f(g(x)) = 
f(u)
g(x) |
Demostración de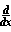
c f(x) = c 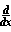f(x)
: partiendo la definación
Dado que:
f(x) = lim(d->0) ( f(x+d)-f(x)
)/d
Resuelva:
c
f(x) = lim(d->0) (c f(x+d)) - (c f(x))/d = c * (f(x+d) - f(x))/d
= c * f(x)
Demostración de
(f(x) + g(x)) =
f(x) + g(x)
: partiendo la definición
Dado que:
f(x) = lim(d->0) ( f(x+d)-f(x)
)/d
Resuelva:
(f(x)
+ g(x)) = lim(d->0) [ (f(x+d) + g(x+d)) - (f(x) + g(x))
] / d
= (f(x+d)-f(x))/d + (g(x+d)-g(x))/d =
f(x) + g(x)
Demostración de la Regla de la Cadena
: f(g(x)) = 
f(g) g(x) :
partiendo la definición
Dado que:
f(x) = lim(d->0) ( f(x+d)-f(x) )/d
Resuelva:
f(g(x))
= df/dx = (f(g(x+d) - f(g(x))/d
df/dx * 1/(dg/dx) = [ (f(g(x+d) - f(g(x))/d ] * [ d/(g(x+d)
- g(x)) ]
= ( f(g(x+d))-f(g(x)) )/(g(x+d)-g(x)) = df/dg
df/dx = df/dg * dg/dx
|
